rempsusnocongeobap.netlify.com

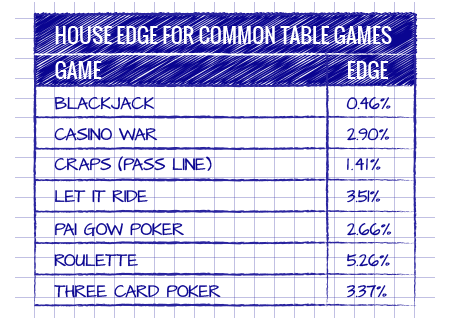
Slot machines Pai Gow Poker Bonus House Edge are popular worldwide for their record-breaking jackpots and exciting themes. With few rules and strategies to consider, slot games are perfect for new casino players. Try some free slot games now or discover online slots to play for real money. In this video, the viewer will learn how to arrange their cards so they have the best house edge. This is also called the “House Way” and these are the rules. The house edge in Pai-Gow Poker depends on whether you’re banking or not. When you are banking and you play by the house way, you’re essentially playing with no house edge. However, when you’re not banking, the house edge is about 2.9%. If the house allows co-banking, then the house edge. The 5Dimes bonus casino offers Pai Gow in which a 2% commission goes to the player, rather than dealer, resulting in a house edge of 0.7%. Microgaming casinos offer an optional bonus bet with a 5.79% house edge. Other softwares that I am aware do not offer side bets. Variance and Bet Size.

1. Pai Gow Poker House Edge Definition
2. Pai Gow Poker House Edge Casino Games

Introduction

EZ Pai Gow Poker is a commission-free pai gow poker variant. Instead of the usual 5% commission, if the dealer has exactly a queen high 5-card hand, then the hand will automatically resolve in a push. In addition, there are four side bets the player may wager on. There is also a fully-wild joker version is available to play in California.

Rules

The rules are the same as conventional pai gow poker, except:

1. There is no commission.
2. If the dealer has a queen-high hand, meaning the poker-value of the best five-card hand is exactly a queen high, the hand will automatically result in a push.
3. Most casinos do not allow player banking. If they do, then the rules revert to normal pai gow poker for that hand.
4. There are various side bets available, described separtely below.

Strategy

My strategy advice is that same as that for conventional pai gow poker. If you don't have time to study it, then if in doubt, ask the dealer to set your hand by the house way.

Analysis

The following table shows the probability and return from each outcome. The lower right cell shows a house edge of 2.47%.

EZ Pai Gow Poker Return Table — Partially Wild Joker

Event	Pays	Probability	Return
Win	1	0.274597	0.274597
Push	0	0.426112	0
Lose	-1	0.299291	-0.299291
Total	1	-0.024694

Side Bets

There are lots of side bets that are available for casino management to tack onto EZ Pai Gow Poker. Here are some that I know of. You won't see all of these at any one table, probably just two or three of them.

Dynasty Bonus

Similar to the Fortune Bonus in conventional pai gow, the Dynasty Bonus pays if the player?s best five-card hand is at least a three of a kind. It does not matter how the player sets his hand. If another player gets a four of a kind or higher, the player will win an 'Envy Bonus.' The following table shows what each hand pays for both the player's hand and the Envy Bonus. The cell bottom row in the second to last column shows a house edge of 8.16%, before considering the Envy Bonus. The cell in the bottom row and last column shows that each additional player lower the house edge by 0.90%, because of the Envy Bonus.

Version 1

In this version the player has to bet only $1 to qualify for the Envy Bonus.

Dynasty Bonus Return Table — Version 1

Event	Dynasty Pays	Envy Bonus	Combinations	Probability	Dynasty Return	Envy Bonus
A-5 Straight Flush + Natural AQ Suited	2000	$100	12	0.000000	0.000156	0.000008
Natural 7-card Straight Flush	2000	$80	32	0.000000	0.000415	0.000017
Wild 7-card Straight Flush	1000	$40	196	0.000001	0.001272	0.000051
Royal Flush + Natural AQ Suited	1000	$60	72	0.000000	0.000467	0.000028
Five Aces	500	$20	1128	0.000007	0.003659	0.000146
Royal Flush	120	$10	26020	0.000169	0.020257	0.001688
A-5 Straight Flush	120	$15	4308	0.000028	0.003354	0.000419
Straight Flush	50	$4	180324	0.001170	0.058492	0.004679
Four of a Kind	25	$1	307472	0.001995	0.049868	0.001995
Full House	5	$0	4188528	0.027173	0.135865	0.000000
Flush	4	$0	6172088	0.040041	0.160165	0.000000
Three of a Kind	3	$0	7672500	0.049775	0.149326	0.000000
Straight	2	$0	11034204	0.071584	0.143168	0.000000
Losing combinations	-1	$0	124556196	0.808056	-0.808056	0.000000
Total	154143080	1.000000	-0.081593	0.009031

The next table shows the overall house edge according to the number of players, including yourself, and various bet amounts. Note that the high edge is lowest at a bet of $1. This is because the win for the Envy Bonus is the same, regardless how much the player bets.

Dynasty Bonus House Edge — Version 1

Players	$1 bet	$2 bet	$3 bet	$5 bet	$10 bet
6	3.64%	5.90%	6.65%	7.26%	7.71%
5	4.55%	6.35%	6.96%	7.44%	7.80%
4	5.45%	6.80%	7.26%	7.62%	7.89%
3	6.35%	7.26%	7.56%	7.80%	7.98%
2	7.26%	7.71%	7.86%	7.98%	8.07%
1	8.16%	8.16%	8.16%	8.16%	8.16%

Version 2

In this version the player has to bet $5 to qualify for the Envy Bonus, which are usually five times as large as the Envy Bonuses in version 1.

Dynasty Bonus Return Table — Version 2

Event	Dynasty Pays	Envy Bonus	Combinations	Probability	Dynasty Return	Envy Bonus
A-5 Straight Flush + Natural AQ Suited	2000	$500	12	0.000000	0.000156	0.000008
Natural 7-card Straight Flush	2000	$400	32	0.000000	0.000415	0.000017
Royal Flush + Natural AQ Suited	1000	$300	72	0.000000	0.000467	0.000028
Wild 7-card Straight Flush	1000	$200	196	0.000001	0.001272	0.000051
Five Aces	500	$100	1128	0.000007	0.003659	0.000146
Royal Flush	120	$75	26020	0.000169	0.020257	0.002532
A-5 Straight Flush	120	$50	4308	0.000028	0.003354	0.000279
Straight Flush	50	$20	180324	0.001170	0.058492	0.004679
Four of a Kind	25	$5	307472	0.001995	0.049868	0.001995
Full House	5	$0	4188528	0.027173	0.135865	0.000000
Flush	4	$0	6172088	0.040041	0.160165	0.000000
Three of a Kind	3	$0	7672500	0.049775	0.149326	0.000000
Straight	2	$0	11034204	0.071584	0.143168	0.000000
Losing combinations	-1	$0	124556196	0.808056	-0.808056	0.000000
Total	154143080	1.000000	-0.081593	0.009735

The next table shows the overall house edge according to the number of players, including yourself, and various bet amounts. Note that the high edge is lowest at a bet of $1. This is because the win for the Envy Bonus is the same, regardless how much the player bets.

Dynasty Bonus House Edge — Version 2

Players	$5 bet	$10 bet	$15 bet	$20 bet	$25 bet
6	3.64%	5.90%	6.65%	7.03%	7.26%
5	4.55%	6.35%	6.96%	7.26%	7.44%
4	5.45%	6.80%	7.26%	7.48%	7.62%
3	6.35%	7.26%	7.56%	7.71%	7.80%
2	7.26%	7.71%	7.86%	7.93%	7.98%
1	8.16%	8.16%	8.16%	8.16%	8.16%

Bonus Bet

This is very similar to the Dynasty Bonus, and is paired with games offering the G3 electronic side bet wagering. As far as I can tell at the Rampart casino, it is just titled the 'Bonus Bet.'

Bonus Bet Return Table

Event	Dynasty Pays	Envy Bonus	Combinations	Probability	Dynasty Return	Envy Bonus
Natural 7-card Straight Flush	8000	$5000	32	0.000000	0.001661	0.000208
Royal Flush + Natural AQ Suited	2000	$1000	72	0.000000	0.000934	0.000093
Wild 7-card Straight Flush	1000	$500	196	0.000001	0.001272	0.000127
Five Aces	400	$250	1128	0.000007	0.002927	0.000366
Royal Flush	150	$50	26020	0.000169	0.025321	0.001688
Straight Flush	50	$20	184644	0.001198	0.059894	0.004791
Four of a Kind	25	$5	307472	0.001995	0.049868	0.001995
Full House	5	$0	4188528	0.027173	0.135865	0.000000
Flush	4	$0	6172088	0.040041	0.160165	0.000000
Three of a Kind	3	$0	7672500	0.049775	0.149326	0.000000
Straight	2	$0	11034204	0.071584	0.143168	0.000000
Losing combinations	-1	$0	124556196	0.808056	-0.808056	0.000000
Total:	154143080	1.000000	-0.077656	0.009268

Pai Gow Poker House Edge Definition

The next table shows the overall house edge according to the number of players, including yourself, and various bet amounts. Note that the high edge is lowest at a bet of $1. This is because the win for the Envy Bonus is the same, regardless how much the player bets.

Bonus Bet House Edge

Players	$1-$4 bet	$5 bet	$10 bet	$15 bet	$25 bet
6	7.77%	3.13%	5.45%	6.22%	6.84%
5	7.77%	4.06%	5.91%	6.53%	7.02%
4	7.77%	4.99%	6.38%	6.84%	7.21%
3	7.77%	5.91%	6.84%	7.15%	7.39%
2	7.77%	6.84%	7.30%	7.46%	7.58%
1	7.77%	7.77%	7.77%	7.77%	7.77%

Protection

Similar to Pai Gow Insurance, the Protection bet pays if the player?s best five-card hand is an ace high or less. The following table shows what each event pays, the probability, and contribution to the return. The lower right cell shows a house edge of 7.01%.

Protection Return Table

Event	Pays	Combinations	Probability	Return
A-5 Straight flush	120	4320	0.000028	0.003363
9 High Pai Gow	100	31080	0.000202	0.020163
T High Pai Gow	25	248640	0.001613	0.040326
J High Pai Gow	15	963480	0.006251	0.093758
Q High Pai Gow	7	2719500	0.017643	0.123499
K High Pai Gow	5	6386940	0.041435	0.207176
A High Pai Gow	3	14430780	0.093619	0.280858
Non-Pai Gow	-1	129358340	0.83921	-0.83921
Total:	154143080	1	-0.070066

Red/Black

The Red/Black wagers pay if the chosen color is in the majority of the player?s cards. In other words, the player needs at least four cards of the chosen side to win. The joker counts as neither color. The following table shows what each event pays, the probability, and contribution to the return. The lower right cell shows a house edge of 2.68%.

Protection Return Table

Event	Pays	Combinations	Probability	Return
7	5	657800	0.004267	0.021337
4 to 6	1	73033740	0.473805	0.473805
0 to 3	-1	80451540	0.521928	-0.521928
Total	154143080	1	-0.026786

Queen's Dragon

The Queen's Dragon pays if the dealer has exactly a queen-high pai gow hand. Winning bets may pay 45 or 50 to 1, depending on how generous/stingy casino management is. The following two return tables show the house edge is 18.84% at 45 to 1, and 10.02% at 50 to 1.

Queen's Dragon — 45 to 1

Event	Pays	Combinations	Probability	Return
Queen-high pai gow	45	2,719,500	0.017643	0.793921
Lose	-1	151,423,580	0.982357	-0.982357
Total	154,143,080	1.000000	-0.188436

Queen's Dragon — 50 to 1

Event	Pays	Combinations	Probability	Return
Queen-high pai gow	50	2,719,500	0.017643	0.882135
Lose	-1	151,423,580	0.982357	-0.982357
Total	154,143,080	1.000000	-0.100222

Progressive Pai Gow

This is a progressive side bet found with G3 electronic betting units. The player may bet $1 to $25. All jackpot wins are the same, regardless of bet size, so I would never bet more than $1. As usual with progressive bets, wins are on a 'for one' basis. That means you don't get your original bet back, even if you win. To be consistent with other pages on this site, the following page is on a 'return basis,' meaning what the player can expect to get back for his bet, based on a $1 bet.

Progressive Pai Gow

Event	Pays	Combinations	Probability	Return
7-card Straight Flush	Jackpot	$228	0.000001	0.000000
Five Aces	0.1×Jackpot	$1,128	0.000007	0.000000
Royal Flush	$500	$26,092	0.000169	0.084636
Straight Flush	$100	$184,644	0.001198	0.119787
Four of a Kind	$75	$307,472	0.001995	0.149604
Full House	$4	$4,188,528	0.027173	0.108692
All other	0	$149,434,988	0.969456	0.000000
Total	154,143,080	1.000000	0.462719

The bottom right corner shows a return of 46.27% on all fixed wins. The value of the progressive is 22.11% for each $100,000 in the meter. To reach 100% the meter would need to be $243,011.06. When I saw this bet at the Rampart casino on March 17, 2011, the meter was at $207,361, for a return of 92.12%. This was probably unusually high, because the Rampart has had a truck with a big sign in the back drive around Summerlin promoting the large jackpot.

Acknowledgement

I would like to thank game inventor Dan Lubin and mathematician Charles Mousseau of Future Sight Gaming for providing me the mathematical analysis report of this game, which this page is based on.

Written by:Michael ShacklefordI don't know if anyone knows the real house edge. PGP is a bitch to analyze without a LOT of computing power, and without taking illegal shortcuts such as assuming that the dealer's hand is dealt from a different deck than the player's hand.
In the worst case scenario analysis, you've got combin(53,7) player hands * 21 ways to split it * combin(46,7) dealer hands = 173,259,639,655,502,400 pairs of comparisons to make (pairs, because you need to compare the 5-card hands as well as the 2-card hands).
Now, in the same way that the number of starting hands in video poker can be reduced from 2,598,960 to 134,459 (for a 52-card deck), the number of starting hands for PGP can be reduced from 154,143,080 to 6,972,147. That brings our total down to 6972147 * 21 * 53524680 = 7,836,820,678,847,160 scenarios to test.
And while there are 21 ways to split a 7-card hand into a 5-card hand and a 2-card hand, not all of them are valid (some are foul). I come up with the following totals for the number of valid ways to split PGP hands:

Valid Ways To Split	Total Hands	Unique Hands
1	4,150,964	200,829
2	4,651,952	236,574
3	3,312,668	158,533
4	30,046,884	1,363,252
5	2,223,576	111,104
6	7,186,780	366,431
7	662,864	33,302
8	1,244,576	62,349
9	2,597,100	129,899
10	56,888,820	2,506,548
11	669,384	33,674
12	521,312	27,000
13	143,564	9,253
14	94,284	5,238
15	26,162,652	1,121,390
16	18,904	1,107
17	18,612	1,034
18	13,440,184	595,591
19	0	0
20	0	0
21	108,000	9,039

(I calculated those totals a long time ago, so I'm not entirely sure if they are accurate, but for now let's assume they are.)
If you take the product of the leftmost and rightmost columns, and sum that product for each row, you come up with 64,977,799 unique hand/split scenarios that need to be tested against every possible dealer hand. This brings the total down to 64977799 * 53524680 = 3,477,915,898,579,320 player vs. dealer scenarios to test.
So in order to calculate the actual house edge, you need to be able to run through those 3.5 quadrillion scenarios before the power goes out... and you better hope that you didn't make a mistake in your house way code or in the handling of the Joker (or forget to make your program display the results when it's done processing 30 years later).
I think it would require a mainframe - or a freakin' cluster of them - to properly calculate this game in a reasonable amount of time without taking 'lossy' shortcuts.About 0.1% to 0.2%. Not that much. Calculating the improvements by an exhastive search/simulator is not the way to go, though a lot of tuning can be done with an algebraic approach and an accurate power ranking table that includes the effect from two-side copies. Also, searching on select multi-path hands (hands that can be played well in either setting, such as two pairs, straight or flush with one or two pairs, and four of a kinds, etc.) can prune the simulator process. It's not that bad.
1. Most of the edge increase can come from properly playing two pair hands properly and very accurately (keeping 7's and 2's together with a KJ or KQ instead of requiring an ace, and keeping All two pairs together with an AK, etc.);

Pai Gow Poker House Edge Casino Games

2. Some comes from properly handling straights and flushes with one pair (Play a pair of Jacks or better with and A-10 or better if the straight or flush has a queen or less top); straight or flushes with two pairs (Play the straight or flush if it has an AK top, or if the two pairs are 6's and 5's or less with a Q or less top); the hand 6543322 is better played as 32/65432 than as 65/33224 or 22/33654, Playing a high straight or flush with an AK top is always better than playing it as any two pairs, even a 'strong' two pair hand.
3. and a tiny amount of improvement comes from properly handling full houses with an AK top (don't split if the pair part are 4's or less) and properly handling four of a kinds, but they are so rare a misplay here means very little. Next to no improvement can come from worrying about how to handle full houses that also have a straight or flush with a pair (hands that always include the joker, and where either play is really never a misplay).
There is a slightly different strategy for banking, than for playing against a banker.
Also important to consider is to eliminate the wins and loses that cancel each other out. In this sense, it is better for the house or banker to win two hands and push four hands, than to win four hands and lose two back - for the same net two wins, especially on a commission-free version where no trivial income is made by 'churning hands.' In one case, two net wins comes from two transactions, where in the other, the two net wins comes from six transactions, four of which were useless.
Getting the extra 0.2% while using a strategy of one page, and is still easy to deal is the holy grail in PGP. For the house, the extra 0.2% can add up to about 2% extra in table hold.I think there are more opportunities in Pai Gow Tiles. For one thing, the banking rules are often more lenient, at least in commercial casinos. Also, a lot of time when you are banking the opposition will discuss their hands before you set yours. I bet you could do very well if you knew Chinese and were a white person, too.
Oops, doing the wrong kind of thinking, again. Sorry, Dan :)Your confession is accepted. Three Our Fathers and two hours of Pai Gow Poker at $25 a hand, no peeking....and a massage at Spring Valley and Valley View with the winnings. Viva Las Vegas.